Mars does not have much of an atmosphere, less than 1% of the pressure on Earth, and mostly carbon dioxide at that. However, there are speculations that Mars may have had a denser atmosphere in the past. There are also a lot of speculations that Mars could be terraformed, and thus obtain a breathable atmosphere.

Back in elementary school, I was taught that Earth is a closed system, that is, (with the exception of space travel), no material is lost from Earth, and no material is added to Earth. I believe this is wrong. Molecules in the Earth's atmosphere occasionaly reach escape velocity, traveling off to outer space. I believe that's the reason our atmosphere hardly contains any hydrogen and very little helium (light molecules reach higher velocities). Similarly, Earth is hit all the time by stuff from outer space (meteorites, comets), so material is added to Earth. There are theories that most of the water on Earth originated from comets.

Anyway, back to Mars. Mars is quite a bit smaller than Earth, so the gravity is quite a bit less. The radius is smaller, so that actually increases gravity at the surface, but that is proportional to the square of the radius. The mass of Mars is proportional to the third power of its radius, so the decrease in gravity outweighs (no pun intended) the increase in gravity. If that is too complicated, just accept that the escape velocity on Mars is approximately 5 km/s, while the escape velocity on Earth is approximately 11 km/s.

Ok, good. So now the question is: would a substantial fraction of a Mars atmosphere boil off, that is, would a substantial fraction of the molecules reach escape velocity? This would mean that the atmosphere would cool off (it's the hotter molecules that fly away). To compensate for this, some heat source would have to replenish the lost energy. This could be a man made process, or a biological process, or a physical process. I don't know what, I'm no expert on these matters. Doesn't really matter though. If the atmosphere would go back to its original temperature, then some of the molecules would escape again, causing the atmosphere to cool off again, etcetera. So, we would need some mechanism to replenish the atmosphere again, preferably something biological.

We're going to make a whole lot of assumptions now. First, we assume that the atmosphere behaves like an ideal gas. Furthermore, we assume that the temperature of a breathable atmosphere will be similar to the temperature of Earth's atmosphere, say 280 Kelvins. Last, we assume that the atmosphere is pure oxygen (O₂). You could probably argue that such an atmosphere would not be ideal for breathing, but hey, it should be survivable. This is not a real scientific analysis anyway...

So, now we need to figure out which fraction of the molecules in the atmosphere would have velocities that exceed the escape velocity. That's easier said than done. I had to dig through my old physics textbooks to find the following.

Uggh! What's up with that? Here it goes.

dn/dv is the number of molecules with velocities between v and v + dv.
N is the number of molecules.
m is the mass of one molecule (5.31*10^-26 kg).
k is Boltzmann's constant (1.3807*10^-23 J K^-1).
T is the (absolute) temperature of the atmosphere (280 K).

Anyway, filling in all the values will allow us to plot a graph of this function. The Y-axis gives the fraction of molecules with velocities between v and v + dv. See picture.

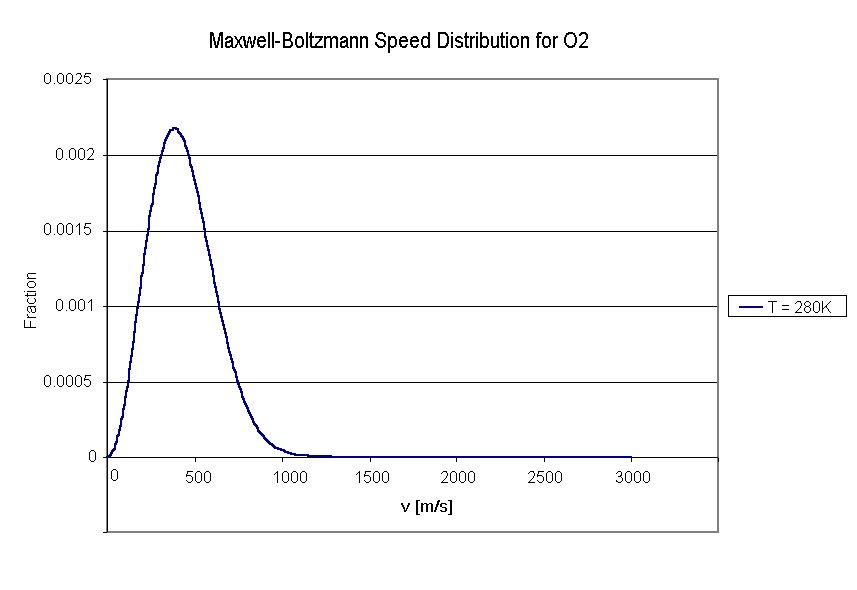
Now, to figure out what fraction of molecules has a velocity higher than the escape velocity, we would have to calculate the integral from the escape velocity to infinity. Unfortunately, the function is a pain in the neck to integrate (has to be done numerically).

We're going to cheat here, and recognize the fact that an extremely small fraction of molecules has velocities higher than 1.5 km/s. Since our escape velocity is > 5 km/s, we can conclude that, yes, Mars should be able to sustain an oxygen atmosphere.

That was easier than I thought. Huh.

There's only one thing that concerns me, though. If you make similar plots for hydrogen (H₂) and helium (He), the same argument applies. Only a very, very small fraction of molecules will reach the escape velocity of Earth (11.2 km/s). So, how did the molecular hydrogen and helium get lost from Earth's atmosphere? In the case of molecular hydrogen, you could speculate that chemical reactions were responsible for this. You can imagine that hydrogen would quickly react with oxygen, and form plain old water (H₂O). However, in the case of helium this does not apply, since the stuff refuses to react with anything.
The only thing I can think of is that the Maxwell-Boltzmann distribution is not valid for helium, since quantum effects play a non-negligible role for light gases (I didn't figure that out myself, I read it). A proper analysis of this would require Fermi-Dirac distributions and Bose-Einstein distributions. I'm going to leave that for now, since these buggers lead to gamma functions and Lerch transcendents and other scary stuff.

Please send comments to webmaster@oldeloohuis.com.